Oscillations and Waves

Oscillatory Motion

Motion of a pendulum is oscillatory.  The pendulum bob rises while it swings to the right, then falls, and again rises as it swings to the left.  In a typical pendulum, like one in a grandfather's clock, this motion repeats over and over again.  Another commonly observed oscillatory motion is the one caused by a spring or other elastic medium.  When we drive our car over a bump, for example, the car oscillates vertically, especially if it has a "bad suspension".  Or when we drop a ball onto a drum skin, the ball bounces up and down repeatedly.  There are many other, perhaps less familiar, oscillatory motions in nature.

What is common to all oscillatory motion is that, after a time, the motion repeats itself. The characteristic time that it takes the motion to make one full cycle is called the period of oscillation.  In most oscillations the value of the period directly depends on the parameters of the oscillator itself.  For example, in the case of the (simple) pendulum, the value of the period depends on the length of the pendulum.  (Exercise: Take a small object, say a door key, and hang it from a string to make a pendulum.  Pull the key to the side, release it, and use your watch to measure the time for 10 of its oscillations.  Next, change the length of this string and repeat this measurement.  From these data determine what adjustment one needs to correct a "fast" grandfather clock!)

The frequency of oscillation is the number of full oscillations in one time unit, say in a second.  A pendulum that takes 0.5 seconds to make one full oscillation has a frequency of 1 oscillation per 0.5 second, or 2 oscillations per second.  The commonly used unit for the number of oscillations per second is the Hertz, so that this same pendulum is said to have a frequency of 2 Hertz, or 2 Hz.

A third measure of oscillatory motion is the maximum distance of travel of the oscillator. This is called its amplitude.  In the case of the pendulum, its amplitude is the maximum height it rises to.  In the case of a spring it is the maximum compression (or stretch) distance.  Notice that to compress a spring further and further, you need to push it harder and harder.  Similarly, to make a swing go higher and higher, you need to give it larger and larger pushes.  So, the amplitude of oscillation is related to the energy of its motion.  The larger the amplitude, the greater the oscillator's energy.

There are two other useful concepts that relate to most oscillators: damping and forcing.  All oscillators that interact with other things around them lose energy.  A pendulum bob that is pulled to the side and released swings a few times and then comes to a stop (typically, its amplitude undergoes a continuous decay) because of friction in the air and in its pivot.  This is called damping.  To make the pendulum swing continuously we need to supply it with repeated "pushes".  This is called forcing it.  So, a real (as opposed to ideal) oscillator can continue to oscillate only so long as forcing is supplied.  It is interesting to note that forcing with different frequencies can have different results, even though the amount of the force may be held fixed.  In fact, in most damped oscillators the maximum amplitude of oscillation is reached when the forcing frequency is chosen to be equal to the natural frequency (in the absence of forcing) of the oscillator. This condition is called resonance and represents the situation in which the maximum energy is transferred from the external agent supplying the forcing to the oscillator.

Questions on Oscillatory Motion

Waves

Mechanical waves are vibrational disturbances that travel through a material medium.  Examples include water waves, sound waves traveling in a material medium such as air or water, waves along a string (as in a musical instrument) or along a steel beam, or seismic waves traveling through the earth.  A general characteristic of all waves is that they travel through a material media (except for electromagnetic waves - discussed later - which can travel through a vacuum) at characteristic speeds over extended distances; in contrast, the actual molecules of the material media vibrate about equilibrium positions at different speeds, and do not move along with the wave.

A wave affects its surroundings as it travels through it carrying energy and momentum.  An example of this that we all have observed is the case of water waves.  When a wave travels on the surface of water it makes floating objects (say leaves, branches, or people floating on the water surface) go up and down.  It is important to note that as a water wave travels in a horizontal direction the floater just goes up and down vertically.  That is to say, the wave does not move the floater with it in its direction of travel.  Another way that energy and momentum get transferred is when objects (i.e. material bodies such as particles, balls, cars, etc.) interact with one another through collisions.    When a billiard ball collides with a second one, momentum and energy are transferred by making the second ball move in the same direction that the first one was moving.  This is quite different from the case of a wave interaction with material bodies.  

Another interesting aspect of waves that is worth noticing is that all that travels is the disturbance.  In the case of water waves, for example, the water itself (droplet/molecule/atom) does not move with the wave.  These aspects of waves are true not only for waves in which the direction of the disturbance (oscillation) is perpendicular to the wave motion, but also for waves in which the oscillation is along the direction of motion of the wave, as in sound waves.   In the case of sound waves, the air molecules do not move with the speed of sound along the wave direction, but remain localized vibrating back and forth along the direction of the the wave travel with very small amplitude.  Water waves and other waves in which their oscillation direction is perpendicular to their travel direction are called transverse, while sound waves and other compression waves in which these directions are the same are called longitudinal.  

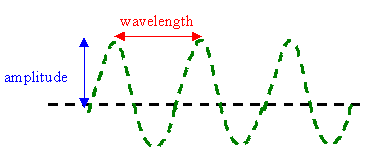
A snapshot of a wave showing its amplitude and wavelength

The vibration caused by a wave, just as a vibration caused by an elastic medium (say, a spring), has an amplitude, a period, and a frequency.  So, a wave also is characterized by its frequency, or period, and its amplitude.  In addition, a wave is also characterized by its speed of travel.  Another way of taking the wave speed into account is to instead refer to the distance that the wave travels to produce a full cycle of its vibration.  This distance is called the wavelength.  As the wave moves by, in a time equal to the period one oscillation of the wave occurs and so the wave has moved along a distance equal to the wavelength.  The velocity of the wave is then given by

since the frequency is the inverse of the period.  In the case of water waves, for example, the distance from the one peak to the next (or one valley to the next) is one wavelength (see the figure just above). Notice that this measure, wavelength, then depends not only on the speed of propagation of the wave, but also on the period (or frequency) of the vibration.

Not only do waves behave very differently from material objects in the context of transmission of momentum and energy, but they also interact with each other differently than material objects do.  When two objects meet each other they collide.  Two waves, on the other hand, do not interact at all but pass "through" each other as ghosts pass through ghosts.  But in the region where the two passing waves overlap the effective disturbance becomes a net sum of the disturbances of the two waves.  So, when a floater happens to be "at the wrong place at the wrong time", i.e. where the peaks of the two waves meet, it experiences a much larger up-down motion.  But were it lucky to be where the peak of one wave meets the valley of another wave of equal amplitude, then it would not move at all; as if there were no waves passing by.  This addition of disturbance, which does not affect the original waves, is purely a wave phenomenon and is called interference.  So, waves do not collide, they interfere.  (Exercise: to see how two waves interfere, work with the applet:  Wave Interference     Try changing the wavelength of the waves and their amplitudes.  One of the interesting results is when the two waves have the same amplitudes and almost the same wavelengths, but not quite.)

Another wave characteristic that has no analog when it comes to travel of material objects is that when a wave reaches an obstacle (or an opening), with dimensions comparable to its wavelength, it bends around the obstacle (and about the opening).  This second wave phenomena is called the diffraction effect.  Check the applet on Wave Diffraction to see how this works.  Try changing the opening size to see how the effects of diffraction sharpen up or get washed out.

Questions on Waves

Electromagnetic Waves

Electrically charged objects attract or repel each other, just as two magnets attract or repel each other.  The electric force that acts between charges has significant differences from the magnetic force that results in the interaction of magnets.  But, for the topic at hand, these forces behave very much the same way in that they are both of the "action-at-a-distance" type.  That is to say, these forces manifest themselves in the absence of any physical contact between the objects that interact with each other.  For example, two magnets exert forces on each other even while they are apart and neither is touching the other.  In fact, the reason that a compass works is that its small magnetized needle rotates because of the magnetic force of the earth, as if manipulated by a ghost.  Another way (model) of explaining this interaction is to state that the earth's magnetic core establishes a magnetic field everywhere in space.  It is this field that affects the compass needle.  This concept of "field" is useful because once we know the field of the earth, then we know how any magnet would be affected once it enters this field.  The field provides an intermediary to understand how action at a distance can work.  With electric charges, each charge produces an electric field which then in turn interacts with other electric charges.

Another advantage of the field concept is that it allows for an easier visualization of what happens when one of the charges (or magnets) begins to move.  In this view, when a charge changes position, the field that it produces also changes in space.  In fact, as the charge oscillates, so does its field.  This oscillating field is what is called an electromagnetic wave.  By making a charge oscillate at one point in space we can cause another charge located further away to undergo oscillatory motion.  Similar to mechanical waves, such as sound and water waves, electromagnetic waves are characterized by their frequency, speed, and amplitude.

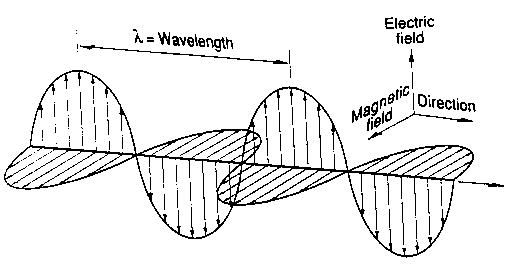
The above picture shows how both the magnetic and electric fields oscillate as the wave propagates to the right.

One interesting aspect of an electromagnetic wave that sets it apart from all other waves we have examined so far is that its propagation requires no medium.  Water waves, which are transverse, of course need water to propagate in.  Sound waves, which are longitudinal, also need a matter medium; although almost any type of matter would do for them (sound travels in air, all known gases, in fluids, in solids, and in plasma, a gas of charged ions).  But oscillating electromagnetic fields travel even in vacuum.  Another interesting feature of electromagnetic waves relates to their speed of propagation.  Mechanical waves travel with a speed that is characteristic of their medium of travel. For example, sound travels much faster in metals than it does in air.  Its speed of travel in air is also dependant on the air pressure, temperature, and humidity.  In the same way, the speed of propagation of electromagnetic waves depends on the material it is passing through, even though it does not "need" the material for its propagation.  This wave travels its fastest in vacuum, with a speed of three hundred million meters per second (300,000,000 m/s or 3x10⁸ m/s) or about 670 million miles per hour.  But what is most significant is that this speed, to our knowledge, is the fastest possible way that nature allows for transmission of energy and momentum.

A third feature of electromagnetic waves that separates it from mechanical waves is that the energy that it propagates also depends on its frequency (of oscillation).  This feature is related to quantization phenomena that we will study in a future topic.  As we will examine later, experimental evidence shows that electromagnetic waves can be represented as if they were made of fixed chunks, or quanta, of traveling oscillations, called photons.  These photons all travel with the same speed

                    photon speed = c = 300,000,000 m/s or 3x10⁸ m/s    (in vacuum),

but each individual photon's energy depends on its frequency, n (Greek letter, called nu).  To be exact, the photon energy, Eg, is just the product of its frequency value, measured in Hertz, with a constant that is named after the German physicist who came up with this idea, Planck.  Planck's constant, denoted by the letter h has a value of 6.63x10^-34 Joule.second; 

    i.e.     photon energy in Joules:       Eg = h n = (6.63x10^-34) (value of photon's frequency in Hz).   

The amplitude of the electromagnetic wave, in this photon picture, is a measure of the number of photons that are traveling together to make up the wave.  So, again, amplitude relates to the energy of propagation. 

Electromagnetic waves, photons, interact with charged entities.  Atoms, molecules, and solids all have charges that vary in quality (sign and degree of binding) and quantity among them.  As a result, electromagnetic waves of different frequency interact differently with the medium through which they propagate.  For purely practical reasons, then, we classify ranges of these frequencies as "bands" and give them specific names.  For example, very high frequency photons (those with frequencies larger than 10²⁰ Hz) are called gamma-rays.  These photons tend to only interact with the atomic nuclei, which are extremely compact in structure.  So, gamma-rays pass through even very thick concrete walls.  X-rays are photons belonging to a band of frequencies anywhere from 10¹⁶ to 10²⁰ Hz.  They can go though material objects too, but they do not have as large a penetration range as the gamma-rays.  For example, X-rays of the frequency used in medicine penetrate through most tissue, except the denser bones or tumors.  All of these ranges of frequencies together is referred to as the electromagnetic spectrum.  Ultraviolet light is invisible to us but is able to give us a sunburn.  Strangely, most uv light is blocked by window glass and so if you drive on a hot summer's day with your arm out the car window, it will get sunburned while full sun on you through the car windshield will not give you a sunburn.  Visible light is just a very narrow band of this spectrum.  Its frequency is of the order of 10¹⁴ Hz.  Our visual system happens to respond to this frequency of photons by generating electric pulses in the visual nervous system, which is interpreted by our brain as sight, as was already discussed.  Note that all the colors that we see are in the narrow visible range of frequencies.  [An interesting tidbit: the word orange was not introduced into the European language until the 10th century when the fruit arrived from the mid-east; orange did not indicate a color until the 1600's.]  Infrared photons and microwave photons have less energy than visible light, but microwaves are able to boil water, while visible light cannot.  This has to do with the specific interactions of different types of electromagnetic waves with matter and will be important in our later discussions on choosing the type of laser to use in various applications. 

 Electromagnetic Spectrum

Questions on Electromagnetic Waves

For further information on Electromagnetic Waves please see the NASA site